Chapter 2 - Design using flexures
Chapter 3 - Design for static stiffness
Large range of motion flexure hinge: Infinity flexure
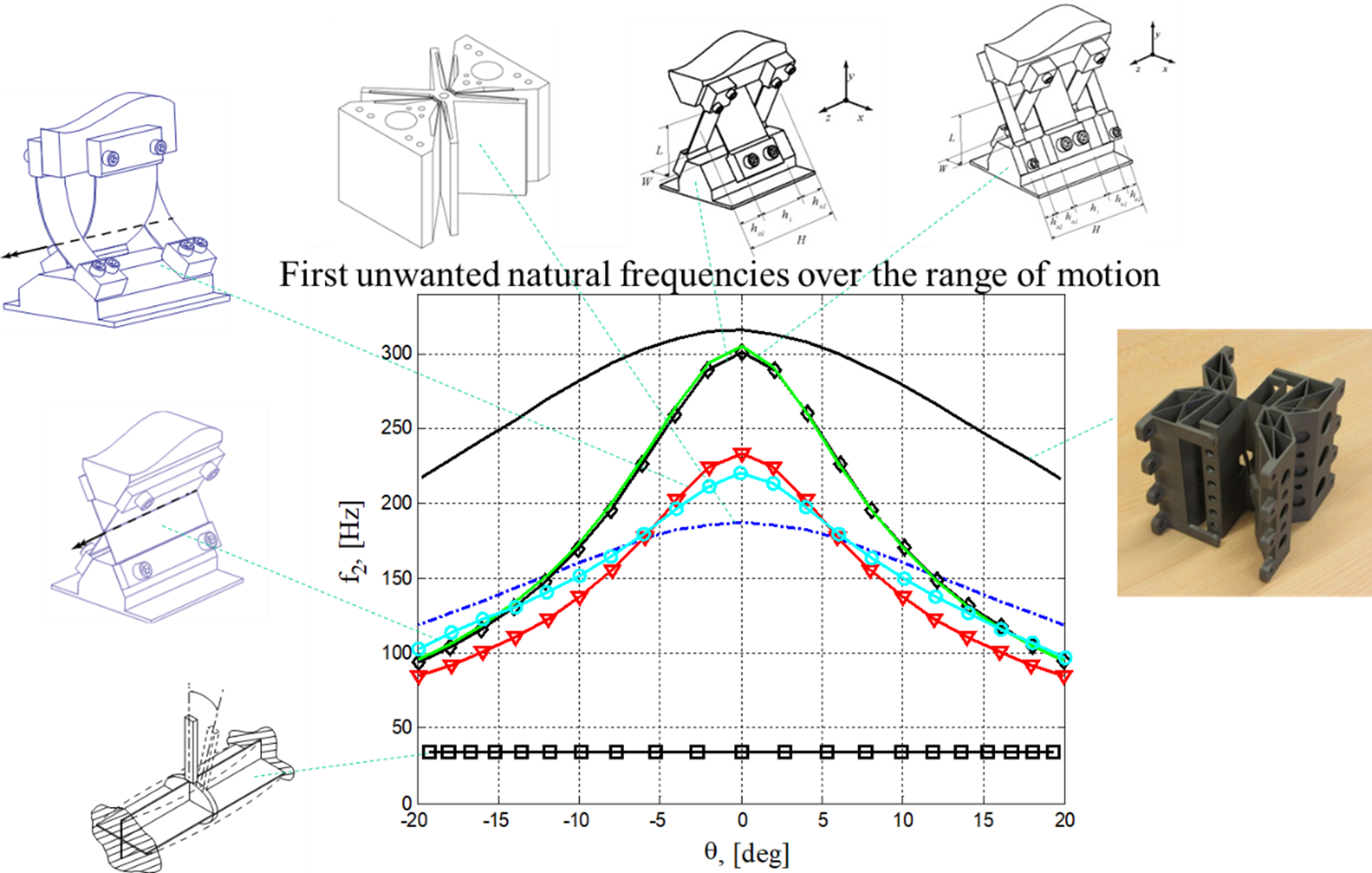
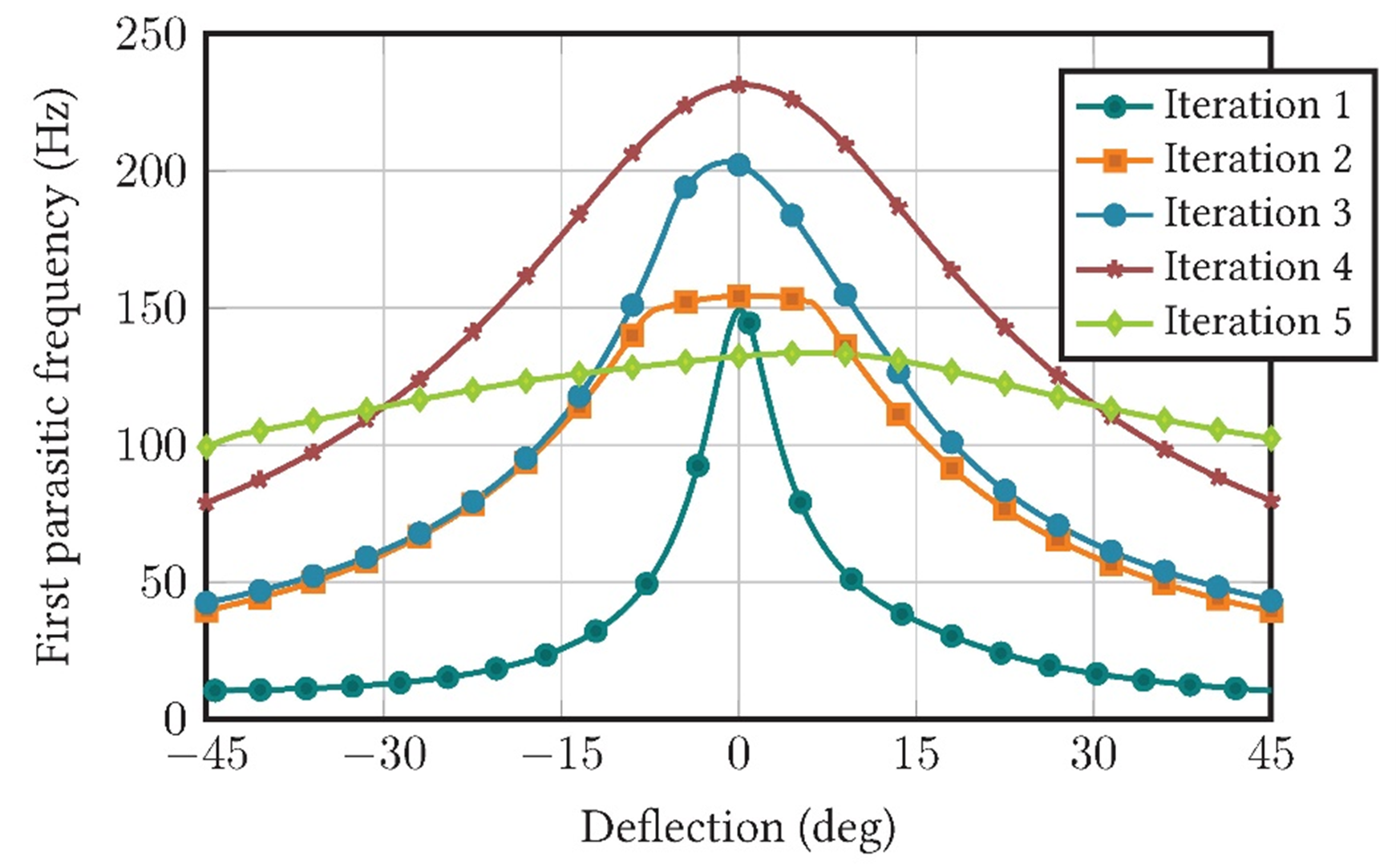
Flexure hinges inherently lose stiffness in supporting directions when deflected, particularly when a large range of motion is required. The infinity flexure is presented which shows a four time increase in support stiffness over a 40 degrees range of motion when compared to the customary designs [1]. An infinity flexure version with 90 degrees range of motion even increases the support stiffness two orders of magnitude compared to a pivot spring flexure optimized for the same load case [2]. Both infinity flexure designs were optimized for maximizing the frequency of the first unwanted vibration mode with a robot arm with payload attached. The eigenfrequency approach ensures all compliance in all directions is taken into account. The numerically predicted natural frequencies and mode shapes have been verified experimentally.
 |  |
| Figure 1. Schematic of the infinity flexure showing the basic leafspring (red) with connected torsional reinforcement, the meander flexure (green). A wire flexure (blue) constrains the lateral motion. | Figure 2. A top view photograph of a titanium printed version. |
 |  |
| Figure 3. Perspective view showing the 40 degrees range of motion. | Figure 4. Perspective view of the infinity flexure for 90 degrees range of motion. The main leafspring is torsionally reinforced by 6 folded leafsprings. The lateral motion is constrained by 2 anti-parallelogram mechanisms in series. |
Principle
A large range of motion flexure hinge can be obtained by using a combination of flexure elements in parallel to create the correct number of constraints (5), additionally stacking such a stage in series to reduce the amount deformation of the flexure elements. The first is common practice the latter is one of the keys to decrease loss of stiffness of flexures when deflected. In its basic form a leafspring is torsionally reinforced using either a folded leafsprings or several leafsprings with a torsionally stiff block in between. A second set is added in series. The combination of parallel and series flexure is called a hybrid flexure.
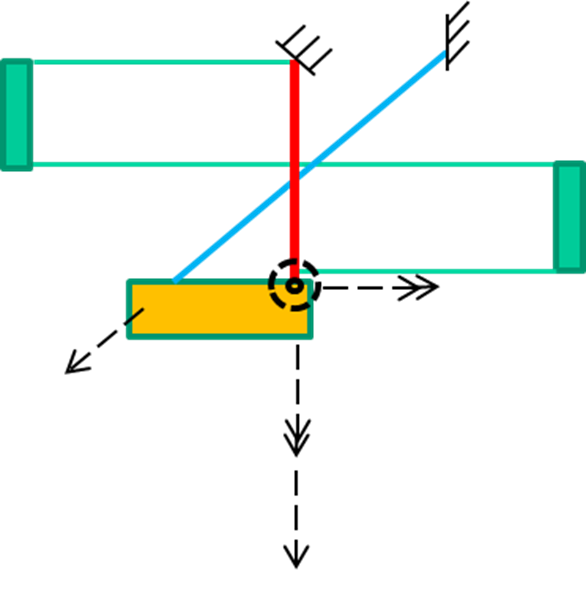
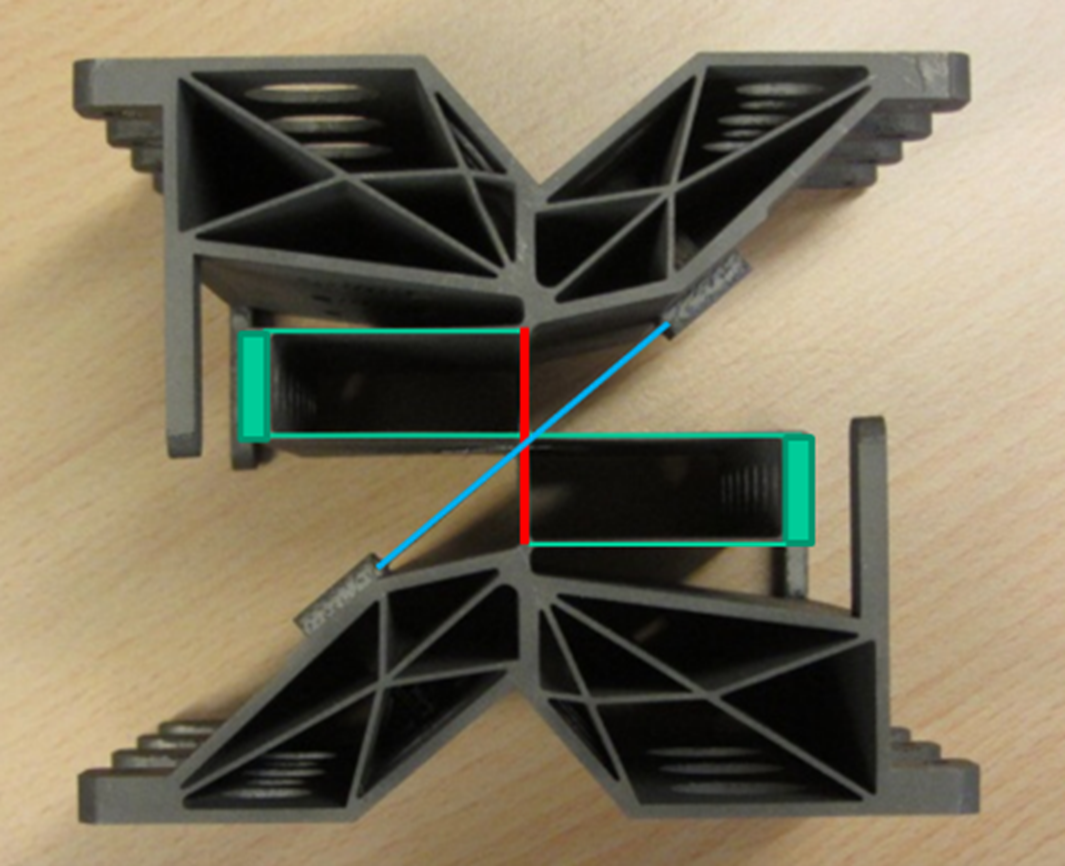
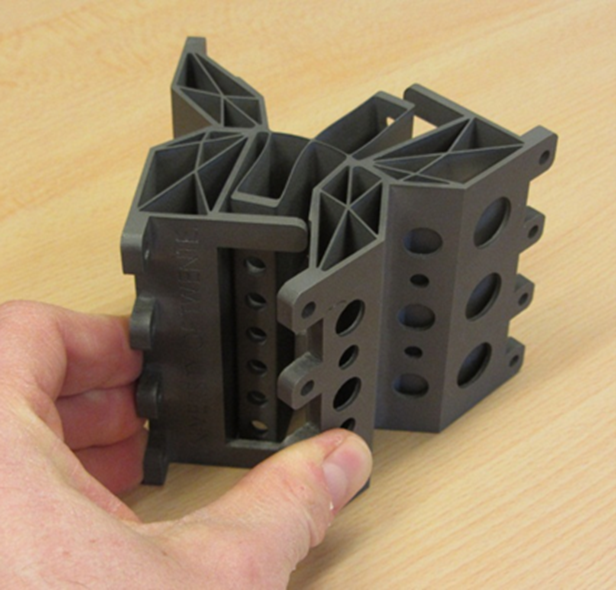
Figure 1 shows a leafspring (red) which is torsionally reinforced by a meander flexure in green, the three leafsprings with torsionally stiff blocks in between [1]. The meander flexure is connected to the red leafspring. A wire-flexure adds a 5th constraint in the lateral direction and is not connected to the leafspring or meander flexure. The second key for large range of motion it that for large displacements the bending stiff element (leaf-spring) is not loaded by torsion due to the connenction with the meander flexure. Vice versa, the torsionally stiff element (the meander flexure) is not loaded by bending for large geometric displacements. This version has shown a range of motion of 40 degrees in total.
A version with 90 degrees range of motion has been shown by Naves [2]. It features a leafspring with a set of 6 folded leafspring around it for torsional reinforcement. The set of 6 ensure again that for large displacements the bending stiff element (leaf-spring) is not loaded by torsion . The constraint of the lateral motion requires more than just a wire flexure. A set of 2 flexure-based anti-parallelogram mechanisms in series constrain the lateral motion with a large longitudinal stiffness over a large range of motion.
 |
| Figure 5. Graph showing several flexure hinge designs including the 40 degrees range of motion infinity flexure joint. Each has been optimized for the same load case. |
 |
| Figure 6. Graph showing several optimized design iterations of the layout for the 90 degrees range of motion infinity flexure joint. Iteration 1 is essentially the cross pivot hinge. Iteration 5 is the 90 degrees range of motion infinity flexure joint. |
Conclusion
The infinity flexures for 40 and 90 degrees range of motion show a increase of about a factor two ten in natural frequency of the first unwanted vibration mode, which represents a huge
improvement in performance. Measurements on the natural frequencies of the
vibrational modes are in good agreement with the modeled results. Hybrid flexures show good stiffness performance over a large range of motion.
Developed by University of Twente
- M. Naves, D.H. Wiersma, S.E. Boer, R.G.K.M. Aarts, D.M. Brouwer
Referentie Bron
[1] D.H. Wiersma, S.E. Boer, R.G.K.M. Aarts, D.M. Brouwer, Design and Performance Optimization of Large Stroke Spatial Flexures, Journal of Computational and Nonlinear Dynamics (ISSN 1555-1415), 9(1), 011016, pp. 10 (2014). DOI: 10.1115/1.4025669
[2] M. Naves, D.M. Brouwer, R.G.K.M. Aarts, Building block based spatial topology synthesis method for large stroke flexure hinges, ASME robotics and mechanisms, 2017, doi: 10.1115/1.4036223.