4.1 Important variables

Biot number

A useful way to determine if a part will experience significant internal thermal gradients is to calculate the Biot number. This number compares the internal heat transfer via thermal conduction to the external heat input.  Divide the external heat transfer coefficient (radiation, convection, thermal contact..) by the internal conduction over the relevant distance (that is, distance from the surface to the interior of the part).

If this number, Bi is:

• much greater than 1, external influences are relatively strong, and the part cannot be expected to maintain a uniform temperature
• much less than 1, internal heat spreading is relatively strong, and the part will have a uniform temperature, even while it heats or cools as a whole.

In the first case, thermal gradients inside the part itself can be significant, and may be calculated as if the external influences are constant (e.g. using the fourier conduction law). In the second case, the part can be considered to have a uniform temperature (but possibly varying in time). Its interaction with its environment (e.g. other parts thermally contacting it) is then analyzed (e.g. using a lumped-capacitance model).

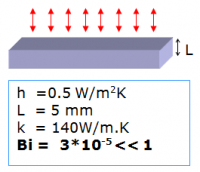
Example 1: thin aluminum plate in vacuum

A thin aluminum plate (excellent internal conduction) in a vacuum is under influence of radiation at room temperature (weak thermal coupling). It will remain very uniform in temperature (low Biot number). Its uniform temperature will vary in time, depending on the part’s total heat capacity and the radiative heat input.

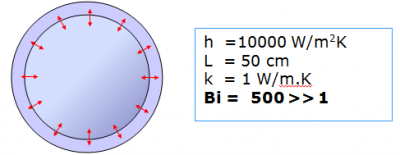
Example 2: 100mm round glass viewing window glued into metal flange

A glass window (glass has poor internal conduction) glued directly in a metal flange (good thermal contact) will heat and cool non-uniformly, and has a high Biot number. The glass temperature will not remain uniform if the flange changes temperature. Thermal gradients in the glass will arise, and may be calculated using e.g. the fourier conduction law, or FEM  software.

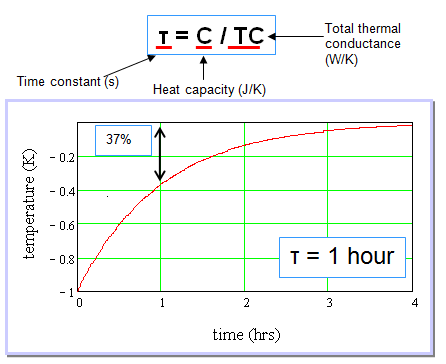
Thermal Time constant

Another useful number is the thermal time constant of a part. By dividing a part’s heat capacity (J/K) by its external thermal contact (W/K), we calculate a time constant (tau). This number gives an idea of the time it will take for a part to adjust to an external  thermal influence. After time tau has elapsed, the part will have heated (or cooled) through 63% of the initial temperature difference between it and the “outside world”.