Radiation
Heat transfer from every object in the form of electromagnetic radiation (or photons). Radiative heat transfer does not require a “medium”, i.e. occurs also in vacuum. The wavelength of the radiation (energy of the photons) depends on the temperature of the object. Objects hotter than 1000K emit radiation at visible wavelengths.
We perceive this as a “red-hot” (ca. 1000K) or even “white-hot” (ca. 5000K) glow. The intensity (but not the wavelength/color) depends on a property called the “emissivity” of a surface. This is defined as compared to a perfect black surface. Surfaces with a high emissivity (near 1) are usually matte, dull and often black surfaces. Shiny or reflective surfaces usually have a low emissivity (near 0).
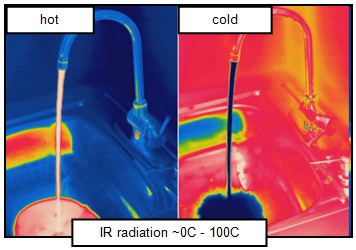
- The type of radiation emitted depends on temperature (see pictures below):
- IR light (0 – 1000K)
- Visible light (1000 – 5000 K)
- UV light (> 5000 K)

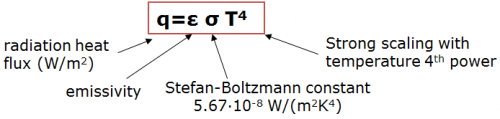
The actual heat flux emitted (or heat loss) from a surface (Watts per square meter of surface area) scales with emissivity (between 0 and 1) and the temperature to the 4th power. The scaling constant sigma is called the Stefan-Boltzmann constant. It is important to use the absolute temperature in Kelvin in this formula.
| Name | Symbol | Units | Description |
| Emissivity | ε | – | Intensity of emitted radiation (ranges from 0 to 1) |
| Stefan-Boltzmann constant | σ | W/(m2K4) | = 5.67·10-8 W/(m2K4) (constant value) relates surface temperature to thermal radiation |
Usually we want to know the heat transfer between two or more surfaces. This is calculated as explained in the in depth part for thermal radiation.
For two surfaces (as shown above), based on the Gebhart factor for three surfaces, Lemmen’s formula to compute the radiative heat transfer rate is complicated already. The denominator is a correction factor for multiple reflections and incorporates the areas of the two surfaces, the emissivities of the surfaces and a “view factor”.
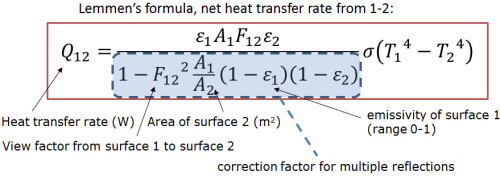
Note: Lemmen’s formula is less accurate when the third surface is highly reflective. In that case the equation below is more accurate to determine the net heat transfer rate.
Warning: in many cases the radiative heat transfer rate for two surfaces within a three or more surface system is calculated using the equation below. However, this equation is only true if the system consists of only two surfaces (F12 = 1).
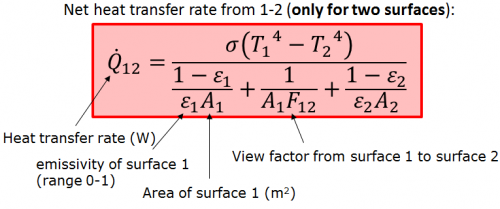
View factor
Imagine you are a fly sitting on a surface. An object floats in the “sky” above your head. What portion of the “sky” is occupied by the object? This is called the view factor. The view factor describes what fraction of a surface’s field-of-view is occupied by the opposing surface.
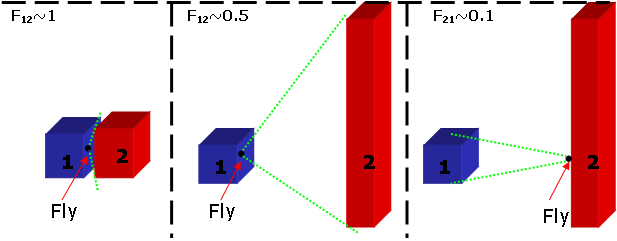
The view factor from surface 1 to surface 2 (F12) can be different than vice-versa (F21). Keep in mind that the view factor is multiplied by the area of the “from” surface. Since the total heat flow from surface 1 to 2 must be equal, but opposite in sign to the heat flow from 2 to 1, this implies that the two view factors F12 and F21 are related. This relationship is given by:
F12A1=F21A2
If all the 1’s and 2’s in the radiation formula are interchanged, the value of Q will remain the same, but opposite in sign, if this view factor relation is used. Pages 543-546 of “A Heat Transfer Textbook” list view factors for various geometries. In addition, the online catalog from “Thermal Radiation Heat Transfer” of Howell& Siegel is a very substantial source of view factors.
Emissivity
Another important factor in the formula is the emissivity of each surface. It’s a number between 0 and 1, indicating how efficiently a surface absorbs and radiates heat. An object with an emissivity of 1 is called a “black body”, since it radiates (and absorbs) heat with perfect efficiency. For non-transparent objects, emissivity is equal to absorption coefficient. Below is a table of typical emissivities for a few materials. Note that surface condition (rough, polished, oxized, etc.) can be as important as the material itself in determining emissivity.
Many more emissivities can be found in:
Page 528 of “A Heat Transfer Textbook”
Electro Optical Industries/hgh-infrared
| Material | Surface condition | Emissivity |
| Aluminium | smooth (shiny) | 0.1 |
| Mu-metal | smooth | 0.15 |
| Silicon | polished | 0.2-0.6 |
| Gold | polished | 0.02 |
| Copper | polished | 0.04 |
| Copper | oxidized | 0.5 |
Radiation – Linearized
The forth order temperature used in the radiation formula, can be linearized as shown below. This is used to compare the radiative heat transfer with the conductive and/or convective heat transfer. The linearization is only valid if the temperature differences are much lower than the absolute temperatures involved. In that case, an average, nominal temperature Tnom is considered constant, while the variable temperature difference is multiplied linearly.
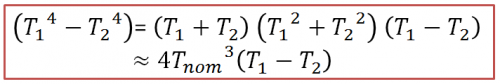
| Name | Symbol | Units | Description |
| Emissivity | ε | – | Intensity of emitted radiation (ranges from 0 to 1) |
| Stefan-Boltzmann constant | σ | W/(m2K4) | =5.67 10-8 W/(m2K4) (constant value) relates surface temperature to thermal radiation |
| View factor | F12 | – | Amount of area a surface occupies in another surface’s Field-of-View (range 0 to 1). The online catalog from “Thermal Radiation Heat Transfer” of Howell& Siegel is a very substantial source of view factors. |
| Nominal temperature | Tnom | K | Nominal temperature (constant value) |
