Conduction
The Fourier's law of heat conduction is a basic law describing a linear conductive heat flux (heat flow per unit area [W/m2]) through a material. Heat flows in the direction opposite to the thermal gradient, thus, from hot to cold. The rate at which this happens is determined by the temperature difference (K) and the thermal conductivity (W/(m K)) of the material.
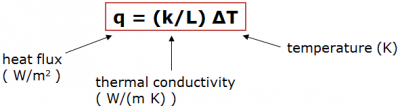
| Name | Symbol | Units | Description |
| Heat flux | q | W/m2 | Heat transfer rate per unit area perpendicular to the heat flow. |
| Thermal conductivity | k | W / (m K) | Material property: describes how easily heat flows through the material. |
Example
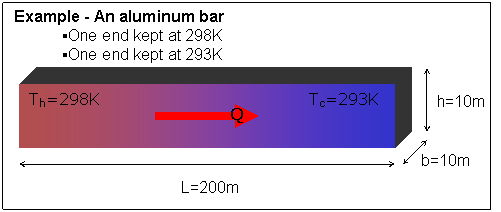
An example is the heat that flows inside an aluminum bar, with ends that are maintained at different temperatures. The gradient is 5K over 200mm, or 25 K/m. Looking up the thermal conductivity of Aluminum, this works out to a heat flux of 4250 W/m2, or 0.425 W over the 10×10 mm2 cross-section of the bar:
- ΔT = 298K – 293K = 5K
- Δx = L = 200 mm
- k = 170 W/(m K) (aluminum)
- Area = b h = 100mm2
q = (k/L) ΔT = 4250 W/m2
Q’ = q Area =0.425 W
Conduction in one direction is often easy to calculate. However, conduction in two and three dimensions can become very difficult to calculate. Therefore a problem is often simplified to 1D. If that is not possible, a more complex numerical computation is an option.

one-dimensional heat conduction